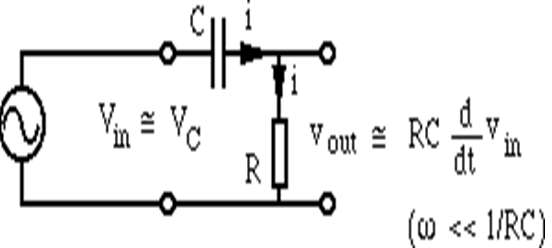
- A differentiator outputs the derivative of the input voltage. A high-pass filter is a differentiator made up of passive components such as resistors and capacitors. The circuit is depicted. Only when the time constant is too small does it act as a differentiator. The output voltage is proportional to the current flowing through the capacitor. C DV / DT is the formula for expressing the current through the capacitor. The output is crossing the resistor. As a result, the output will be RC DV / DT. As a result, input is differentiated.
- The circuit is called a differentiator because its effect is very similar to the mathematical function of differentiation, which means finding a value that depends on the rate of change of some quantity (mathematically). A differentiator circuit’s output wave is ideally a graph of the rate of change of the voltage at its input. The output of a differentiator is proportional to the rate of change of its input, and the actions of the high-pass filter and the differentiator are identical.
- An alternating current source with voltage Vin(t) is connected to an RC series circuit. The voltage across the resistor is the output this time. This time, we only consider low frequencies 1/RC, so that the capacitor has enough time to charge up until its voltage is nearly equal to that of the source.
![]()
- The use of resistors and capacitors on the input and feedback loops of operational amplifiers allows for more accurate integration and differentiation. These amplifiers can also add, subtract, and multiply voltages. An analog computer is a collection of such circuits that can solve simultaneous, differential, and integral equations quickly.
- A square wave input signal is converted or ‘differentiated’ by the Differentiator circuit into high-frequency spikes at its output.
- Kirchhoff’s law is used to demonstrate the relationship between the waveforms across the resistor and capacitor in a series network. The graphical sum of the voltage waveforms in a closed loop must equal the applied waveform because the sum of the voltage drops in a closed loop must equal the total applied voltage. The diagram below depicts a differentiator circuit with the output routed through a variable resistor.
Short Time-Constant Differentiator
- The time constant of the circuit is 10 microseconds when the variable resistor is set to 1,000 ohms and the capacitor value is 0.01 microfarad. The circuit is a short time-constant circuit because the input waveform has a duration of 100 microseconds.
- The voltage across the capacitor is zero at the first instant of time in the short time-constant circuit. A maximum voltage is developed across the resistor as current flows through it. This is depicted in the graph of the figure below at the first instant of time.

- As the capacitor begins to charge, the voltage developed across the resistor begins to fall. The voltage developed across the resistor will have decreased by 63.2 percent of the applied voltage at the end of the first time constant. Because 100 volts is applied, the voltage across the resistor after 1 is 36.8 volts. The voltage across the resistor will be 13.5 volts after the second time constant.
- VR will be 5 volts at the end of the third time constant and 2 volts at the end of the fourth time constant. The voltage across the resistor will be very close to 0 volts at the end of the fifth time constant. Because the time constant is equal to 10 microseconds, charging the capacitor and stopping current flow in the circuit will take a total of 50 microseconds.
- The slope of the charge curve will be very sharp, as shown in the figure above. Until the end of 100 microseconds, the voltage across the resistor will remain at 0 volts. At that point, the applied voltage abruptly drops to zero, and the capacitor discharges through the resistor. The discharge current will be at its peak at this point, causing a large discharge voltage to develop across the resistor. This is represented by the negative spike in the graph above. Because the current flow from the capacitor, which is now acting as a source, is decreasing exponentially, so will the voltage across the resistor.
- In 5 time constants, the resistor voltage will decrease exponentially to 0 volts. This entire discharge action will take 50 microseconds. The discharge curve is also depicted in the above figure. The action resumes at the end of 200 microseconds. In this short time-constant circuit, the output waveform taken across the resistor is an example of differentiation. Positive and negative spikes are produced in the output when the square wave is applied. The rate of change of the input square wave is approximated by these spikes.
Long Time-Constant Differentiator
- The differentiator output will look more like the input if the time constant for the RC differentiator circuit is increased to make it a long time-constant circuit. The circuit’s time constant can be changed by increasing the value of capacitance or resistance. The time constant in this circuit will be increased by increasing the resistance value from 10,000 to 100,000 ohms. Increasing resistance results in a time constant of 1,000 microseconds. The time constant is equal to ten times the length of the input pulse. The figure below depicts the output of this long-time-constant circuit.
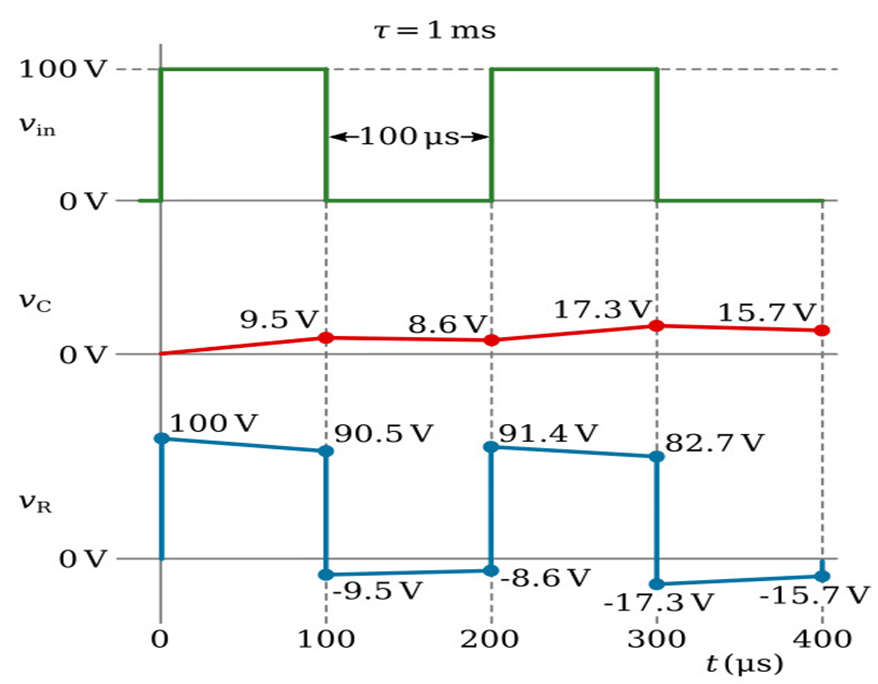
- At the first instant of time, a pulse of 100-volts amplitude with a duration of 100 microseconds is applied. Since the capacitor cannot respond instantaneously to a change in voltage, all of the applied voltage is felt across the resistor. As time progresses, the capacitor will charge and the voltage across the resistor will be reduced. Since the time that the capacitor is permitted to charge is 100 microseconds, the capacitor will charge for only 1/10 of 1τ or to 9.5 percent of the applied voltage. The voltage across the resistor must be equal to the difference between the applied voltage and the charge on the capacitor (100 – 9.5 volts), or 90.5 volts.
- The applied voltage suddenly drops to 0 volts at the end of the first 100 microseconds of input, a 100-volt change. Because the capacitor cannot respond to such a rapid voltage change, it becomes the source of 9.5 volts. In the first instant of time, a -9.5 voltage is felt across the resistor. The voltage across the two components is now equal to zero volts.
- The capacitor discharges over the next 100 microseconds. The voltage across the resistor decreases at the same rate as the capacitor discharges, keeping the total circuit voltage constant at zero. During the second 100 microseconds of operation, the resistor voltage decreases exponentially. The capacitor will now discharge 9.5 percent of its charge, resulting in an 8.6-volt reading. The resistor voltage will rise in a positive direction to -8.6 volts at the end of the second 100 microseconds to keep the total circuit voltage at 0 volts.
- The input voltage suddenly rises to 100 volts at the end of 200 microseconds. Because the capacitor cannot respond to a 100-volt change instantly, the change occurs across the resistor. This step-by-step procedure will be repeated until the circuit stabilizes. After many cycles, the capacitor voltage varies by equal amounts above and below 50 volts. The resistor voltage varies by the same amount above and below 0 volts.
- The RC networks described in this section can also be used as coupling networks. The output of an RC circuit used as a coupling circuit is taken from across the resistor. A long time-constant circuit is typically used. If the applied signal is non-sinusoidal, this will result in an integrated wave shape across the capacitor. In a coupling circuit, however, the signal across the resistor should and will closely resemble the input signal if the time constant is sufficiently long. The voltage across the resistor closely resembles the input signal, as shown in the diagram in the figure above.
- Consider the effects of applying a pure sine wave to a long-time-constant RC circuit (R is much greater than XC). A significant portion of the applied voltage would be developed across the resistor and only a minor portion across the capacitor.
Applications of RC Differentiator
- In the PID controllers.
- As a high pass filter.
- To generate narrow pulses in response to any sharp change in the input signal in wave-shaping circuits.
